MAGNETOTELLURIC ANALYSIS: USE OF
INVARIANCES IN MOHR CIRCLES
1NGUYỄN THÀNH VẤN, 2LÊ VĂN ANH CƯỜNG
Department of
Geophysics, University
of Natural Sciences,VNU-HCM,
Hồ Chí Minh City
Abstract: The magnetotelluric analysis is one of the
methods used in the research on inhomogeneity of 2D and 3D electric
environments, whose depths are about tens kilometers. Explaining
magnetotelluric data is to get useful arbitrary parameters from a general
magnetotelluric impedance tensor. Invariances are drawn from different methods
to express the characteristics of 1D, 2D, and 3D models.
I. INTRODUCTION
The
fundamental model of MT method is created by Tikhonov and Cagniard. In the
model, MT wave transfers into a horizontally stratified earth bed, and the
relation is expressed by:
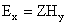 ;
;  (1)
(1)
with
Z: Tikhonov-Cagniard general impedance describing electrical conductivity
distribution versus depth z.
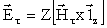 (2)
(2)
where:  and
and 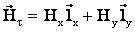 (3)
(3)
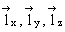 : Unit vectors in Cartersian axes, direction
: Unit vectors in Cartersian axes, direction 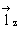 goes down.
goes down.
General
MT impedance Z is considered to be the relation between components 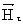 and
and 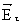 .
.
In
the 1950s, the experiment environment in which Berdichevsky and Cagniard
established impedance Z is a horizontally electrical stratified earth case.
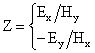 (4)
(4)
However,
because real environments are usually electric nonlayered, general impedance is
considered as a tensor built from 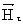 and
and 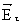 in order to improve
MT method.
in order to improve
MT method.
Berdichevsky
and Zdanov [1] said that there was an invariant relation between MT components,
which expressed electrical conductivity distribution in the earth. It is an
algebraic relation:
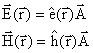 (5)
(5)
where 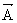 is an arbitrary
vector, which has properties of inducing field and affecting field,
is an arbitrary
vector, which has properties of inducing field and affecting field, 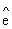 and
and 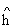 are operators
dependent on electrical conductivity distribution of environment and frequency.
If they can be converted mutually,
are operators
dependent on electrical conductivity distribution of environment and frequency.
If they can be converted mutually, 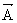 is a matrix to define
linear relation between components of field.
is a matrix to define
linear relation between components of field.
II. PROPERTIES OF GENERAL IMPEDANCE TENSOR
Its
property is dependent on kinds of model. 1D structure, 2D structure and 3D
structure are examined in turn.
- 1D structure: Electrical conductivity distribution only varies through the depth.
The model of Cagniard is 1D structure. In this model, equations Zxx
= Zyy = 0 and Zxy = −Zyx = Z are always right
in arbitrary measured axes.
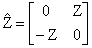 (6)
(6)
The components Zxy and Zyx
of general impedance tensor are related with the change of electrical property
versus depth, but Zxx and Zyy are related with the change
of electric property versus horizon.
- 2D structure: Electrical conductivity distribution varies through depth and a
horizontal axis such as x-axis or y-axis. The horizontal axis, which has
conductance 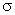 =const, is called as the homogenous axis. Polarized
electromagnetic field is divided into two parts:
=const, is called as the homogenous axis. Polarized
electromagnetic field is divided into two parts:
1.
Parallel (//) or polarized electric field 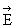 (in case the electric
field is polarized along homogenous axis).
(in case the electric
field is polarized along homogenous axis).
2.
Perpendicular (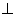 ) or polarized magnetic field
) or polarized magnetic field 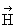 (in case the polarized
magnetic field is perpendicular to homogenous axis).
(in case the polarized
magnetic field is perpendicular to homogenous axis).
 and
and 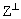 are respectively
parallel and perpendicular components of general impedance tensor. Therefore,
it has zero element on the diagonal.
are respectively
parallel and perpendicular components of general impedance tensor. Therefore,
it has zero element on the diagonal.
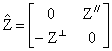 (7)
(7)
- 3D structure: Electrical conductivity distribution varies through depth and
horizontal axes such as x-axis and y-axis. From diversity of these structures,
people use symmetric cylinder structure in which general impedance tensor is
simplest. Assuming that the direction of x-axis is the direction of symmetric
axis, Zr and Zt are centripetal and tangential components
of general impedance tensor. It means that the general impedance tensor has
zero elements on the diagonal:
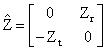 (8)
(8)
3D
symmetric cylinder structure and 2D structure are same in the shape of general
impedance tensor.
III. MOHR CIRCLES METHOD
The
Mohr circles method, most commonly met in the analysis of mechanical stress, is
used to depict magnetotelluric impedance information, taking the real and
quadrature parts of magneto-telluric tensors separately.
Tensor 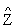 =
=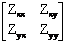
Components
of rotating tensor 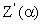 :
:
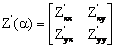
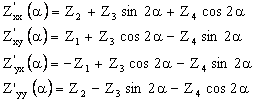 (9)
(9)
where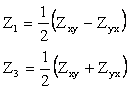
 (10)
(10)
Complex
A = Ar+iAq, where Ar and Aq are,
respectively, real and quadrature. Therefore, we extract circle equations for
real and quadrature parts from the components of the tensor 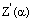 :
:
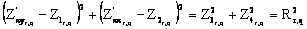 (11)
(11)
where
 (12)
(12)
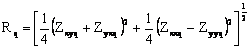 (13)
(13)
A
plot of Z’xx against Z’xy, as the measuring axes rotate,
then describes a circle, where Z’xx and Z’xy are the
values that would be measured with axes rotated 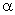 clockwise from those
that gave the initial values of Zxx and Zxy. Similarly,
the components Z’xy and Z’yy also form the relation
through a circle equation.
clockwise from those
that gave the initial values of Zxx and Zxy. Similarly,
the components Z’xy and Z’yy also form the relation
through a circle equation.
Invariants
with rotation of the measured axes: because Z1 and Z2 are
invariants, the distance from the origin of axes to the circle center is
invariant, which is called “central impedance” [3]. Denoting the central
impedance by ZL, its value is given by:
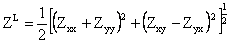 (14)
(14)
Other invariants are the values of the circle
radii Rr and Rq.
If the circle is offset from the Z’xy-axis,
the offset can be expressed by an angle 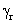 or
or  . The value of tan
. The value of tan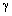 is showed:
is showed:
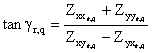 (15)
(15)
Now, we consider the relation between Mohr
circles and the 1D, 2D and 3D structures.
- 1D structure: 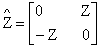 , the circle reduces to its central point, which is on the
horizontal Z’xy-axis.
, the circle reduces to its central point, which is on the
horizontal Z’xy-axis.
- 2D structure: the circle is centered on the horizontal axis, and cut the horizontal
Z’xy-axis at two values. 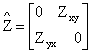
- 3D structure: the circle center moves off the axis, and the invariant 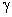 is different to zero.
For highly anisotropic data (either 2-D or 3-D), the circumference of the
circle approaches the origin of the axes (Lilley, 1993a).
is different to zero.
For highly anisotropic data (either 2-D or 3-D), the circumference of the
circle approaches the origin of the axes (Lilley, 1993a).
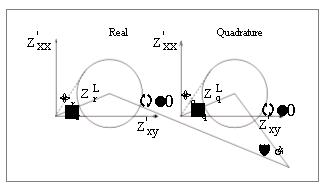
Figure 1. A pair of real and quadrature circles for a tensor, showing the set of
invariant.
From
inspection of the previous figure (Fig. 1), one straight-forward set can be
seen to be:
1.
ZL, the distance from the origin of axes to the circle center, which
gives the 1-D “scale” of a matrix, and is its 1-D kernel.
2.
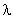 , an angle which gives a measure of the two dimensionality or
anisotropy of a matrix, defined as:
, an angle which gives a measure of the two dimensionality or
anisotropy of a matrix, defined as: 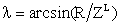
3.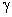 , an angle which gives a measure of the three dimensionality
of a matrix; ZL,
, an angle which gives a measure of the three dimensionality
of a matrix; ZL, 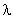 , and
, and 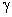 have values for both
the real and quadrature matrices of the
tensor, and thus comprise six invariants.
have values for both
the real and quadrature matrices of the
tensor, and thus comprise six invariants.
A
seventh invariant, which is a further parameter of three dimensionality, links
the real and quadrature parts of a tensor and may be expressed as: 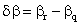 ; where the angle
; where the angle 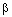 is between the
horizontal axis and the line linking the circle center to the observed point.
is between the
horizontal axis and the line linking the circle center to the observed point. 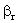 and
and 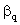 refer to the values of
refer to the values of 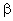 for the real and
quadrature parts of a tensor, respectively.
for the real and
quadrature parts of a tensor, respectively.
Example:
3D impedance tensor
(a)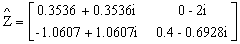
(b)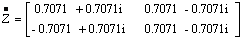
From the Fig. 2. the Mohr
method shows the 3D structures of the environments. In Fig. 2. (b), although
the quadrature Mohr circle shows 2D structure, the structure is 3D (because, in
the real part, the invariant 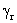 is different to zero).
is different to zero).
IV. ASSESSMENT
Simons
Spritz’s approach gives less information (6 parameters). The Eggers’s
parameters are rather similar to La Torraca and Yee’s (8 parameters) but the
beginning ideas to establish them are different. The principal axes of
polarization ellipses of eigenvector pair (Ei, Hi) are
perpendicular in Eggers’s method (the biorthogonal method). On the other hand,
in the La Torraca and Yee‘s method, the principal axis directions for the  and
and  vectors are not at right angles as in the biorthogonal
analysis. Because of invariant phases of eigenvalues of tensor
vectors are not at right angles as in the biorthogonal
analysis. Because of invariant phases of eigenvalues of tensor 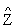 in Eggers’s method
while phases of eigenvalues of tensor
in Eggers’s method
while phases of eigenvalues of tensor 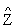 in La Torraca and
Yee’s method are variant (although they vary little), Eggers’s method is more
effective. The Mohr circles construction (Lilley’s method) gives seven
invariants of a tensor which explain the properties of 1D, 2D and 3D
environment clearly and easily understandable. To research Mohr circles method
further, we use it to analyze 3D model data order to draw helpful geological
information.
in La Torraca and
Yee’s method are variant (although they vary little), Eggers’s method is more
effective. The Mohr circles construction (Lilley’s method) gives seven
invariants of a tensor which explain the properties of 1D, 2D and 3D
environment clearly and easily understandable. To research Mohr circles method
further, we use it to analyze 3D model data order to draw helpful geological
information.
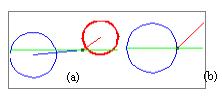
Figure 2. Real (bold line)
and quadrature (thin line) Mohr circles in the 3 D examples.
- 3-D magnetotelluric
modelling: A 3-layer model whose first layer contains 3D conductivity masses is
researched at frequency 2700 Hz. The 3D conductivity masses are ellipse-shaped
mass and sphere mass. The ellipse-shaped mass has major axis a = 50 km, minor
axis b = 12.5 km, conductivity Sc of ellipse center and conductivity
So of the ellipse outer. The sphere mass has radius r = 5 km and
conductivity of the sphere mass St. The distance between the center
of sphere mass and the major axis of ellipse-shaped mass is 7.5 km, and the
distance between the center of sphere mass and the minor axis of ellipse-shaped
mass is 5 km.
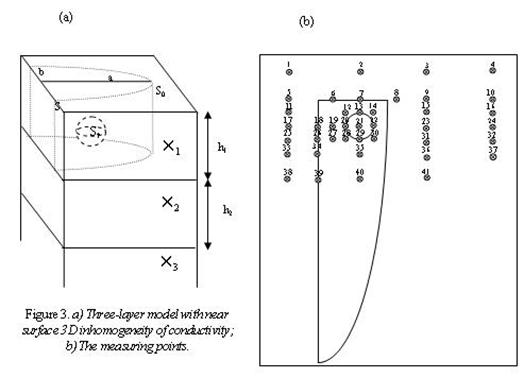
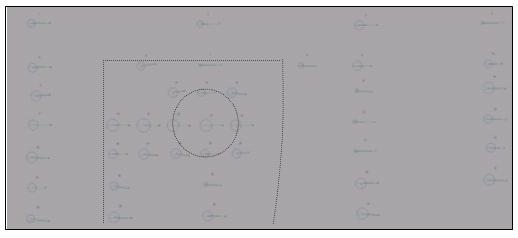
Figure. 4. Real (bold) and quadrature (thin) Mohr
circles in the 3D model.
The 3D model: Resistivities of three layers are, respectively: 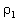 =100
=100 ;
; 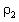 =1000000
=1000000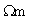 ;
;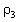 =1
=1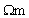 ;
;
Conductivity
Sc of the ellipse center: Sc =10 CM;
Conductivity
S0 of the ellipse outer: S0
= 10 CM;
Conductivity
St of the sphere mass: St
= 100 CM;
The
depths of the first layer and the second layer:
h1 = 1 km; h2 = 200 km.
1. Analysis of the 3d model:
- Mohr circles method:
At
the measuring points 1-5, 7-11, 13, 15-26, 29, 31-33, 35-37, 39 and 40, the
Table 1. The set of invariant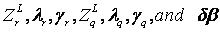 of the 3D model.
of the 3D model.
|
STT
|
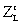
|
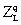
|
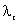
|
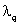
|

|
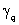
|
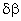
|
|
1
|
0.000015
|
0.000203
|
18.03023
|
12.90871
|
1.859049
|
1.547317
|
2.125996
|
|
2
|
0.000015
|
0.000205
|
13.40122
|
9.593889
|
0.572939
|
0.720012
|
1.235592
|
|
3
|
0.000018
|
0.000271
|
16.13138
|
15.26543
|
0.502306
|
0.338899
|
1.079667
|
|
4
|
0.000013
|
0.000184
|
7.56186
|
4.486621
|
1.775164
|
1.290494
|
11.61628
|
|
5
|
0.000015
|
0.000209
|
19.8633
|
14.7104
|
1.162498
|
0.932863
|
0.992373
|
|
6
|
0.000015
|
0.000209
|
20.11097
|
16.15612
|
7.789108
|
6.256836
|
6.457061
|
|
7
|
0.000013
|
0.000183
|
5.066163
|
3.262733
|
2.015711
|
1.448568
|
31.09535
|
|
8
|
0.000015
|
0.000214
|
12.43617
|
10.04313
|
-3.56432
|
-3.24952
|
3.627354
|
|
9
|
0.000034
|
0.000516
|
21.54779
|
19.78241
|
-1.21088
|
-1.14076
|
0.030031
|
|
10
|
0.000016
|
0.000212
|
22.79522
|
17.28933
|
1.064586
|
0.874946
|
0.837864
|
|
11
|
0.000017
|
0.000229
|
28.482
|
23.23413
|
5.909015
|
4.77612
|
2.404132
|
|
12
|
0.000019
|
0.000265
|
29.36073
|
25.06058
|
9.340026
|
7.76614
|
2.746595
|
|
13
|
0.00002
|
0.000291
|
19.08616
|
16.07348
|
0.763432
|
0.495173
|
0.759273
|
|
14
|
0.000019
|
0.000274
|
23.11654
|
20.36288
|
-6.58996
|
-6.03368
|
0.752431
|
|
15
|
0.000021
|
0.000317
|
8.02095
|
6.979198
|
-3.84067
|
-3.52215
|
2.313074
|
|
16
|
0.000034
|
0.000508
|
22.26512
|
20.4194
|
-2.42938
|
-2.32048
|
0.174716
|
|
17
|
0.000016
|
0.000211
|
21.61166
|
16.49809
|
-0.36782
|
-0.1184
|
0.634357
|
|
18
|
0.000016
|
0.000215
|
28.10045
|
22.1313
|
-0.57066
|
-0.51298
|
0.060401
|
|
19
|
0.000017
|
0.000227
|
31.7643
|
26.14267
|
-0.75517
|
-0.77698
|
0.164174
|
|
20
|
0.000022
|
0.000306
|
25.92506
|
22.06239
|
-0.14975
|
-0.2335
|
0.257288
|
|
21
|
0.000028
|
0.000401
|
23.8888
|
21.41278
|
0.093607
|
0.028576
|
0.175762
|
|
22
|
0.000022
|
0.000317
|
22.00075
|
19.50587
|
-0.1715
|
-0.24801
|
0.485068
|
|
23
|
0.000022
|
0.000321
|
6.467167
|
5.682756
|
-1.06338
|
-1.00927
|
0.590644
|
|
24
|
0.000029
|
0.000439
|
15.3243
|
13.95562
|
-1.99235
|
-1.89829
|
0.109096
|
|
25
|
0.000035
|
0.000521
|
20.21577
|
18.54821
|
-2.22631
|
-2.14347
|
0.160561
|
|
26
|
0.000016
|
0.00021
|
24.91441
|
19.1862
|
-1.63166
|
-1.15395
|
1.016015
|
|
27
|
0.000017
|
0.000226
|
29.22115
|
23.64501
|
-7.06008
|
-6.02628
|
2.084077
|
|
28
|
0.000019
|
0.000262
|
27.33654
|
23.11352
|
-9.84447
|
-8.49831
|
2.557173
|
|
29
|
0.000021
|
0.000295
|
19.46554
|
16.59716
|
-0.8483
|
-0.89335
|
0.26702
|
|
30
|
0.00002
|
0.00028
|
24.92287
|
22.26862
|
5.900111
|
5.160111
|
1.135459
|
|
31
|
0.000021
|
0.000312
|
4.807794
|
4.528576
|
1.679112
|
1.480348
|
3.761664
|
|
32
|
0.000013
|
0.000514
|
43.99519
|
20.01832
|
-5.67866
|
-2.18032
|
36.65731
|
|
33
|
0.000015
|
0.000207
|
24.54191
|
19.07863
|
-0.64937
|
-0.02775
|
1.411758
|
|
34
|
0.000015
|
0.000205
|
20.42065
|
15.95597
|
-9.40079
|
-8.20674
|
4.797871
|
|
35
|
0.000013
|
0.000191
|
10.00696
|
7.048838
|
-3.77989
|
-3.97125
|
11.31574
|
|
36
|
0.000017
|
0.000245
|
21.83839
|
19.17688
|
2.25696
|
1.449625
|
3.142483
|
|
37
|
0.000035
|
0.000521
|
20.18581
|
18.5102
|
-3.11462
|
-2.99428
|
0.363877
|
|
38
|
0.000013
|
0.000178
|
16.64263
|
12.6226
|
-7.33216
|
-7.20092
|
1.191505
|
|
39
|
0.000014
|
0.000189
|
23.8599
|
18.13984
|
-2.361
|
-1.93338
|
1.287537
|
|
40
|
0.000013
|
0.000183
|
21.25694
|
16.7339
|
-2.25421
|
-1.8297
|
1.172901
|
|
41
|
0.000026
|
0.00039
|
21.73615
|
20.01137
|
-6.5198
|
-5.98825
|
0.047091
|
invariants 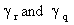 are quite small (
are quite small (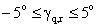 ), radii of real and quadrature Mohr circles are nearly
zeros. The centers of the Mohr circles are in the horizontal axes Zxy.
Therefore, they show 1D, 2D structures of the model environment. To explain
this, we consider locations of the measuring points expressing the affect of
the sphere and ellipse-shaped anomalies. The points 5, 7-10, 18, 26 and 39 are
in the axis of ellipse mass. The points 17, 19-24, 2, 13, 29, 35 and 40 are in
the axis of sphere mass. The points 1, 3, 4, 11, 15, 16, 25, 31-33, 36 and 37
are far from two masses.
), radii of real and quadrature Mohr circles are nearly
zeros. The centers of the Mohr circles are in the horizontal axes Zxy.
Therefore, they show 1D, 2D structures of the model environment. To explain
this, we consider locations of the measuring points expressing the affect of
the sphere and ellipse-shaped anomalies. The points 5, 7-10, 18, 26 and 39 are
in the axis of ellipse mass. The points 17, 19-24, 2, 13, 29, 35 and 40 are in
the axis of sphere mass. The points 1, 3, 4, 11, 15, 16, 25, 31-33, 36 and 37
are far from two masses.
At
the measuring points 6, 12, 14, 27, 28, 30, 34, 38, and 41, the invariants 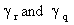 change onsiderably
change onsiderably 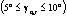 . The centres of the Mohr circles move off the horizontal
axes Zxy and the
. The centres of the Mohr circles move off the horizontal
axes Zxy and the 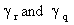 differ from zeros. The
inhomogeneity of the environment is expressed clearly. Again, locations of the
measuring points express the affect of the sphere and ellipse-shaped anomalies
to explain the inhomogeneity. The points 6, 27, 34, 38, and 41 sit near the
boundary of two anomaly masses. Especially, the 3D points 12, 14, 28, and 30
affected by sphere mass and the 2D points 13, 20, 22 and 29 create boundary of
two anomaly masses ( in the Tab. 1, the bold and italic rows are used for the
points 12, 14, 28 and 40; the bold rows are used for the points 13, 20, 22 and
29.).
differ from zeros. The
inhomogeneity of the environment is expressed clearly. Again, locations of the
measuring points express the affect of the sphere and ellipse-shaped anomalies
to explain the inhomogeneity. The points 6, 27, 34, 38, and 41 sit near the
boundary of two anomaly masses. Especially, the 3D points 12, 14, 28, and 30
affected by sphere mass and the 2D points 13, 20, 22 and 29 create boundary of
two anomaly masses ( in the Tab. 1, the bold and italic rows are used for the
points 12, 14, 28 and 40; the bold rows are used for the points 13, 20, 22 and
29.).
When
the biorthogonal method is applied to analyze the 3D model data, the result
also makes the sphere mass outstanding. In the measuring points 12, 14, 28 and
30, the directions of the red bold 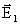 are directed toward
the sphere mass. In the measuring points
13, 20, 22, and 29, the linear polarized ellipses show 1D and 2D structures of
the model. Therefore, the measuring points 12, 14, 28, 30, 13, 20, 22, and 29
create a boundary of two anomaly masses (Fig. 5.).
are directed toward
the sphere mass. In the measuring points
13, 20, 22, and 29, the linear polarized ellipses show 1D and 2D structures of
the model. Therefore, the measuring points 12, 14, 28, 30, 13, 20, 22, and 29
create a boundary of two anomaly masses (Fig. 5.).

Figure 5. E-polarization ellipses for both eigenstates
in the 3D model.
E1(red bold line); E2(blue line).
V.
CONCLUSION
By
considering two real and quadrature elements, the Mohr circles method gives the
useful approach to analyze the magnetotelluric data. With information gotten
from the set of seven Mohr circle parameters, we have enough evidences to
affirm an 1D, 2D and 3D environment, then, continue to measure or choose data
to establish a reserve model problem.
REFERENCES
1. Berdichevsky M.N., Dmitriev V.I., 1992. Magnetotelluric
sounding of horizontally homogeneous media. Moscow (in Russian).
2. Berdichevsky M.N., Nguyen Thanh Van., 1991. Magnetovariational
vector. Izv. Akad. Nauk USSR, Fizika Zemli, 3 : 52-62. Moscow.
3. Lilley F.E.M., 1998. Magnetotelluric tensor decomposition: Part I.
Theory for a basic procedure. Geophysics,
63 : 1885 -1897. Part II. Examples of a basic procedure. Geophysics, 63 : 1898-1907.
4. Nguyễn Thành Vấn, 1996. The methods of assessment and classification of
the geoelectrical inhomogeneities in magnetotelluric data processing. J. of
Sci. and Techn., 4 : 48-52. Hà
Nội (in Vietnamese).
5. Nguyễn Thành Vấn, 2005. Magnetotelluric tensor: Decomposition and
applications. Sci. and Techn. Dev. 8/8 : 26-34. VN Nat. Univ.-HCM City.
6. Nguyễn Thành Vấn, Lê Vân Anh Cường, 2008. Magnetotelluric
analysis: Mohr circles. Sci. and Techn.
Dev., 11/11 : 5-11. VN Nat. Univ.-HCM City.
![]() ;
; ![]() (1)
(1)![]() (2)
(2)![]() and
and ![]() (3)
(3)![]() : Unit vectors in Cartersian axes, direction
: Unit vectors in Cartersian axes, direction ![]() goes down.
goes down.![]() and
and ![]() .
.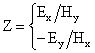 (4)
(4)![]() and
and ![]() in order to improve
MT method.
in order to improve
MT method.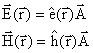 (5)
(5)![]() is an arbitrary
vector, which has properties of inducing field and affecting field,
is an arbitrary
vector, which has properties of inducing field and affecting field, ![]() and
and ![]() are operators
dependent on electrical conductivity distribution of environment and frequency.
If they can be converted mutually,
are operators
dependent on electrical conductivity distribution of environment and frequency.
If they can be converted mutually, ![]() is a matrix to define
linear relation between components of field.
is a matrix to define
linear relation between components of field.![]() (6)
(6)![]() =const, is called as the homogenous axis. Polarized
electromagnetic field is divided into two parts:
=const, is called as the homogenous axis. Polarized
electromagnetic field is divided into two parts: ![]() (in case the electric
field is polarized along homogenous axis).
(in case the electric
field is polarized along homogenous axis).![]() ) or polarized magnetic field
) or polarized magnetic field ![]() (in case the polarized
magnetic field is perpendicular to homogenous axis).
(in case the polarized
magnetic field is perpendicular to homogenous axis).![]() and
and ![]() are respectively
parallel and perpendicular components of general impedance tensor. Therefore,
it has zero element on the diagonal.
are respectively
parallel and perpendicular components of general impedance tensor. Therefore,
it has zero element on the diagonal.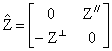 (7)
(7)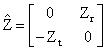 (8)
(8)![]() =
=![]()
![]() :
: ![]()
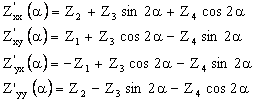 (9)
(9)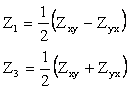
 (10)
(10)![]() :
: ![]() (11)
(11) (12)
(12)![]() (13)
(13)![]() clockwise from those
that gave the initial values of Zxx and Zxy. Similarly,
the components Z’xy and Z’yy also form the relation
through a circle equation.
clockwise from those
that gave the initial values of Zxx and Zxy. Similarly,
the components Z’xy and Z’yy also form the relation
through a circle equation. ![]() (14)
(14)![]() or
or ![]() . The value of tan
. The value of tan![]() is showed:
is showed: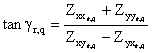 (15)
(15)![]() , the circle reduces to its central point, which is on the
horizontal Z’xy-axis.
, the circle reduces to its central point, which is on the
horizontal Z’xy-axis. 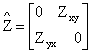
![]() is different to zero.
For highly anisotropic data (either 2-D or 3-D), the circumference of the
circle approaches the origin of the axes (Lilley, 1993a).
is different to zero.
For highly anisotropic data (either 2-D or 3-D), the circumference of the
circle approaches the origin of the axes (Lilley, 1993a).



