COMPUTATION OF WAVE FIELD IN THE ĐỒNG
TRANH ESTUARY, CẦN GIỜ BY USING WAVE
REFRACTION MODEL
VÕ LƯƠNG HỒNG PHƯỚC, NGUYỄN ĐỨC TOÀN,
ĐẶNG TRƯỜNG AN, TRƯƠNG CÔNG HẠNH
University of
Natural Sciences, Vietnam National University,
Hồ Chí Minh City,
227 Nguyễn Văn Cừ, Dist. 5, HCMC. vlhphuoc@phys.hcmuns.edu.vn
Abstract: The Đồng Tranh
River, the longest of main rivers in
Cần Giờ Mangrove Biosphere Reserve, connects the mangrove forests to the East Việt Nam Sea. In recent years, some coastal
mangrove areas at the Đồng Tranh estuary are being eroded seriously. Waves,
tidal currents, rainfall or river flow changes have been considered as the main
reasons for the erosion in this area. In this study, the wave field of the
studied area at the Đồng Tranh estuary is computed by using the wave refraction
mode. The model is based on the irrotationality of wave number and conservation
of the wave action. The model includes wave-current interaction and energy
dissipation due to wave breaking in tidal, shallow-water zone. Based on the
data of mean wave characteristics, mean currents and real bathymetry at the
observed area, the calculated results of the wave field distribution at the
Đồng Tranh estuary are given. The change of wave fields depends strongly on
initial wave characteristics, currents and water depth of the observed area.
They also prove that, the concentration of high wave energy in some sites could
be considered as the main cause of erosion in surrounding mangrove areas,
especially at Nang Hai mangrove forest. The results also indicate that the
model provides a realistic solutions in many practical problems, when some
sophisticated models are not available.
I. INTRODUCTION
The Đồng Tranh
River, with a length of
67.50 km, is the longest of main rivers in the Cần Giờ District, south of Hồ
Chí Minh City. Many studies have been proving that the dynamic processes in
this river, as well as the sedimentation and erosion mechanism in its mouth
zone, are complicated and changeable. The changes are presumably caused by
various natural conditions, such as sedimentary changes, changes of wave
propagation, tidal currents, rainfall or river flow change, and by human
impact, such as development of shrimp ponds, deforestation [5, 6, 8, 9]. The serious erosion at Nang Hai mangrove area in the
Đồng Tranh estuary has been proved as due to wave action [8]. However, up to
now the wave field in the Đồng Tranh estuary has not been studied yet. Therefore,
this paper aims to calculate the wave field at the Đồng Tranh estuary by using
the wave refraction model. This model is based on the irrotationality of wave
number and conservation of the wave action. The model includes wave-current
interaction and energy dissipation due to wave breaking in tidal, shallow-water
zone.
II.
THEORETICAL MODEL FOR WAVE REFRACTION
The refraction model is
based on the idea of irrotationality of wave number and conservation of the
wave action. The irrotationality of wave number is usually expressed in form of
the Snell’s law [2]:
 (1)
(1)
Where: k is wave number and
q: wave direction with respect to the x-axis.
The dynamic part of the
problem is described by the conservation of wave action. In order to develop a
quantitative model for waves at least on a macroscopic level, the energy
dissipation due to wave breaking is included in the equation of the
conservation of wave action as follows [4]:
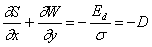 (2)
(2)
Where: S and W are the x, y
components of the wave action;
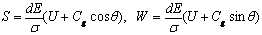 (3)
(3)
Where: the dE is the
incremental of wave energy over the wave number band dk and at a directional
angle q; the D is wave action dissipation due to wave
breaking.
To estimate the dissipated
wave action D due to wave breaking, the resemblance between surf zone waves and
bores is applied from [3].
The wave motion in the
coastal area is induced by wind wave incident from the open sea along the
offshore boundary. The mean wave height 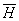 and mean wave
direction
and mean wave
direction 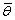 are assumed to be
known along this boundary. A coastal boundary is a moving boundary due to
tides. Within the area of interest, the water depth h(x, y) is a varying
function of the both coordinates. Moreover, the current velocity vector
are assumed to be
known along this boundary. A coastal boundary is a moving boundary due to
tides. Within the area of interest, the water depth h(x, y) is a varying
function of the both coordinates. Moreover, the current velocity vector 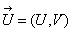 is assumed to be
known. To avoid the difficulties in defining the boundary condition at the
lateral boundaries, outside the area the bottom topography is defined by
parallel bottom contours. Unknown functions of the problem are characteristic
wave parameters, i.e. significant wave height Hs, mean wave length
is assumed to be
known. To avoid the difficulties in defining the boundary condition at the
lateral boundaries, outside the area the bottom topography is defined by
parallel bottom contours. Unknown functions of the problem are characteristic
wave parameters, i.e. significant wave height Hs, mean wave length 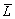 and mean wave
direction
and mean wave
direction 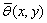 in the grid points.
in the grid points.
For the arbitrary bottom
configuration, the equations (1) and (2) can be solved only numerically. In
this study, the finite-difference scheme suggested by Dalrymple [1] is used. In
this case, a central difference in x and averaged forward and backward
differences in y provides for an O (Dx2, DxDy, Dy2)
error.
III.
CALCULATIONS OF WAVE FIELD AT THE ĐỒNG TRANH ESTUARY
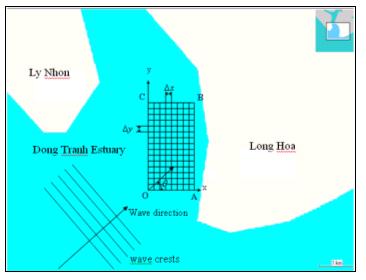
1. Location of the studied site:
The studied arrea is
situated at the Đồng Tranh estuary, Cần Giờ District, south of Hồ Chí Minh City.
The Cần Giờ District lies in a recently formed, soft, silty delta with an
irregular, semi-diurnal tidal regime. The Đồng Tranh is a calm area except for
the eastern side near the Đồng Tranh estuary, from the Đồng Hoa River
to the Khe Đôi creek. The Đồng Tranh area is less affected by strong
wind-induced waves as it is sheltered by the Lý Nhơn and Long Hòa Capes, either side of the estuary. These
capes show a trend to be extended due to the alluvial deposition process. As a
result, wave propagation into the Đồng Tranh River
can change direction from time to time and high waves do not propagate into its
estuary all year round. Only winds from the SE to SSW can create direct waves
propagation to Đồng Tranh. During the northeast monsoon with easterly
disturbance, waves propagation to Đồng Tranh become high, especially for
ENE-ESE wind. The waves in Đồng Tranh estuary are also strong, if there is some
wind from the SE, SSE or S, in the
transitional season from April to early May. The southwestern monsoon can not
create high waves in the Đồng Tranh estuary, except for the case when there is a tropical storm generating SE wind
in summer near the offshore of South Việt Nam [7].
2. Dimension of chosen area and initial input
data:
The
selected area for wave field calculation is from the Đồng Hoa River
to the Khe Đôi creek. The coordinate system O(x,y,z) and the calculated grid
OABC are chosen as shown in Fig. 1. The
dimension of the area is about 1300×870 m, with the grid space of 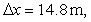
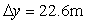 .
.
The
geographical positions of calculated area OABC are as follows: O = 10o22’50”
N, 106o52’4.6” E; A = 10o22’49.8” N, 106o52’33.56”
E; B = 10o23’49.2” N, 106o52’34” E; C = 10o23’49.2”
N, 106o52’4.6” E. Based on
the measured data on the Đồng Tranh River [7], the initial conditions for wave
characteristics are chosen as follows: wave period T = 3s, wave direction q = 45°, mean velocity 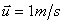 in the NE direction
over the observed area. The initial wave height will be chosen in two cases:
mean wave height (H0 = 0.5 m) and high wave height (H0 =
1 m).
in the NE direction
over the observed area. The initial wave height will be chosen in two cases:
mean wave height (H0 = 0.5 m) and high wave height (H0 =
1 m).
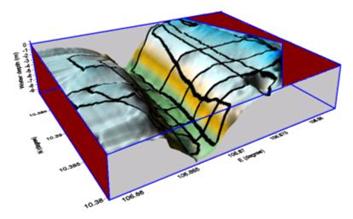
The topography at the Đồng Tranh River
was measured in 2008, as shown in Fig. 2. Because of tide on the Đồng Tranh River
is rather high, about 2-4 m, the water level should be chosen so that the water
level at the muddy flat in front of the sea-mangrove boundary must be high
enough for model calculation. The height difference between the muddy flat and
the deepest site of the Đồng Tranh estuary is about 10 m. Three cases for
high-, medium- and low-water levels have been considered. Consequently, the
water levels at the muddy flat can get 1.5, 1.0 and 0.5 m.
IV. COMPUTED RESULTS OF WAVE FIELD AT THE ĐỒNG
TRANH ESTUARY
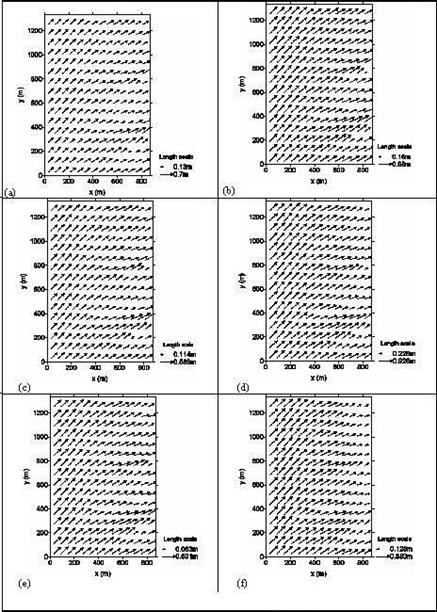
The Fig. 3 and 4 show the computed results of wave
fields (Fig. 3) and of significant wave heights (Fig. 4) when the water levels
are high (a, b), medium (c, d) and low (e, f) and when initial wave heights get
0.5 m (a, c, e) and 1 m (b, d, f). It is obvious that the waves get refracted
and transformed as propagating into shallower area. The wave directions change
as they travel from the estuary to shallower water. The approaching angles
decrease and the wave crests tend to conform to the bottom contours. Results of
q(x, y) calculation are relevant to Snell’s law. The wave height at any particular point depends on two
basic mechanisms, i.e. wave shoaling and energy dissipation. At relatively
great water depth, the wave shoaling dominates and wave height is increasing.
When the limiting water depth is reached, the waves start to be broken and wave
height decreases. When the water levels are high and medium (cases a, b, c),
most of wave heights increase and the waves are not broken. However, when the
water level is low enough (cases e, f) or the initial wave height is high
enough (case d), the breaking waves can be observed. Especially in the case f,
when the water level is low as well as the initial wave is high, most of waves
are broken and the wave height decreases quickly. It is obvious that the wave
field depends strongly on initial wave characteristics, currents and water
depth of the observed area.
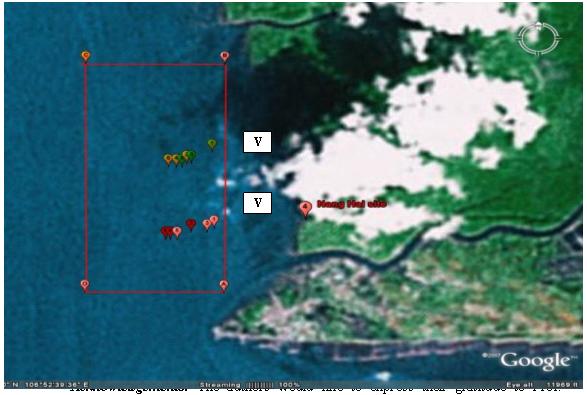
From the computed results of wave field at the Đồng Tranh estuary in six
different cases, it should be noted that many highest wave heights concentrate
mainly in two special sites, namely X and Y as shown in Fig. 5. In the area X,
the highest waves are found in all six cases while in the area Y, the highest
waves can be seen only in case of low water. In the Fig. 5, it can be seen that
the area X is not far from the Nang Hai mangrove forest. The Nang Hai area is
being eroded seriously and quickly, proving that wave action is the main reason
for such erosion [7, 8]. Therefore, the calculated results for the
concentration of high-wave energy in the area X can be explained and emphasized
that wave energy is one of the most important reason of the serious erosion at
Nang Hai site.
Acknowledgements: The
authors would like to express their gratitude to Prof. Massel S.R. and Prof. La
T. Cang for invaluable advices in the development of the wave model.
REFERENCES
1. Dalrymple R.A., 1988. Model for refraction
of water waves. J. of Waterway, Port.,
Coastal and Ocean. Eng.,
114/4 : 423-435. ASCE.
2. Massel S.R., 1989. Hydrodynamics of coastal zones. Elsevier Sci. Publ. Comp., Amsterdam.
3. Massel S.R., Belberova D.Z., 1990. Parameterization of
the dissipation mechanism in the surface waves induced by wind. Arch. Mech., 42 : 515-530.
4. Massel S.R.,
Naguszewski A., 1991.
Model for refraction and dissipation of water waves. Proc. Conf. Coastal and Ocean. Eng., 129-134.
5. Mazda Y., Magi M., Nanao H., Kogo M., Miyagi T., Kanazawa N., Kobashi D., 2002. Coastal erosion due to long-term
human impact on mangrove forests. Wetl.
Ecol. and Manag., 10 : 1-9.
6. Victor S., Neth L., Golbuu Y., Wolanski E., Richmond R.H., 2006. Sedimentation in mangroves and coral reefs in a wet tropical
island, Pohnei, Micronesia. Est., Coast. and Shelf Sci., 66 : 409-416.
7. Vo Luong Hong Phuoc, 2006. Surface waves propagation in mangrove forest
and induced suspended sediment concentration. PhD. Thesis, Inst. of Ocean., Sopot,
Poland.
7. Vo Luong H.P., Massel S.R., 2006. Experiments
on wave motion and suspended sediment concentration at Nang Hai, Cần Giờ
mangrove forest, Southern Vietnam. Oceanologia, 48/1 : 23-40.
9. Wolanski E., Mazda Y., Ridd P., 1992. Mangrove hydrodynamics. In Robertson A.I., Alongi D.M. (Eds).
Tropical mangrove ecosystems. Amer. Geoph. Union, Washington,
43-62.
![]() (1)
(1)![]() (2)
(2)![]() (3)
(3)![]() and mean wave
direction
and mean wave
direction ![]() are assumed to be
known along this boundary. A coastal boundary is a moving boundary due to
tides. Within the area of interest, the water depth h(x, y) is a varying
function of the both coordinates. Moreover, the current velocity vector
are assumed to be
known along this boundary. A coastal boundary is a moving boundary due to
tides. Within the area of interest, the water depth h(x, y) is a varying
function of the both coordinates. Moreover, the current velocity vector ![]() is assumed to be
known. To avoid the difficulties in defining the boundary condition at the
lateral boundaries, outside the area the bottom topography is defined by
parallel bottom contours. Unknown functions of the problem are characteristic
wave parameters, i.e. significant wave height Hs, mean wave length
is assumed to be
known. To avoid the difficulties in defining the boundary condition at the
lateral boundaries, outside the area the bottom topography is defined by
parallel bottom contours. Unknown functions of the problem are characteristic
wave parameters, i.e. significant wave height Hs, mean wave length ![]() and mean wave
direction
and mean wave
direction ![]() in the grid points.
in the grid points.![]()
![]() .
.![]() in the NE direction
over the observed area. The initial wave height will be chosen in two cases:
mean wave height (H0 = 0.5 m) and high wave height (H0 =
1 m).
in the NE direction
over the observed area. The initial wave height will be chosen in two cases:
mean wave height (H0 = 0.5 m) and high wave height (H0 =
1 m).